Unlocking the Mystery: What is Inverse Addition?
Have you ever encountered the term inverse addition and wondered what it means? Whether you're a math enthusiast, a student, or someone looking to enhance their problem-solving skills, understanding inverse addition is crucial. This concept, often overlooked, plays a significant role in mathematics and real-world applications. In this post, we'll break down the mystery of inverse addition, explore its importance, and provide practical examples to help you grasp it effortlessly. (Mathematical Concepts, Problem-Solving Skills, Real-World Applications)
What is Inverse Addition?
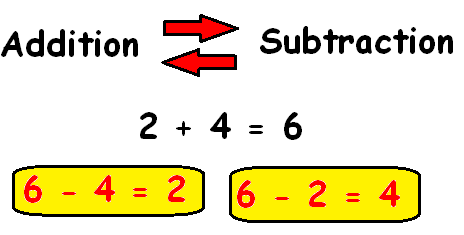
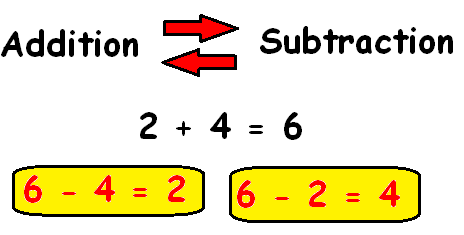
Inverse addition refers to the operation that “undoes” addition. Just as subtraction is the inverse of addition, inverse addition involves finding the value that, when added to a number, returns it to zero. Mathematically, if you have a number ( a ), its additive inverse is ( -a ), because ( a + (-a) = 0 ). This concept is foundational in algebra and forms the basis for understanding more complex mathematical operations. (Algebra Basics, Mathematical Operations)
Why is Inverse Addition Important?

Inverse addition is not just a theoretical concept; it has practical applications in various fields. From balancing equations in chemistry to managing finances, understanding additive inverses helps in solving real-world problems efficiently. It also lays the groundwork for advanced topics like linear algebra and calculus. (Practical Applications, Advanced Mathematics)
- Problem Solving: Simplifies complex equations.
- Financial Management: Helps in budgeting and balancing accounts.
- Scientific Research: Essential for accurate calculations in experiments.
How to Apply Inverse Addition

Applying inverse addition is straightforward. Follow these steps:
- Identify the Number: Start with the number you want to find the inverse for.
- Change the Sign: Convert the number to its opposite (e.g., ( 5 ) becomes ( -5 )).
- Verify the Result: Add the original number and its inverse to ensure the sum is zero.
📌 Note: Inverse addition works for all real numbers, including fractions and decimals.
| Number | Additive Inverse | Sum |
|---|---|---|
| 7 | -7 | 0 |
| -3.5 | 3.5 | 0 |
| 1/2 | -1/2 | 0 |

Checklist: Mastering Inverse Addition
- Understand the definition of inverse addition.
- Practice finding additive inverses for various numbers.
- Apply inverse addition in real-world scenarios.
- Explore advanced applications in algebra and calculus.
Inverse addition is a simple yet powerful concept that unlocks a deeper understanding of mathematics. By mastering it, you'll not only improve your problem-solving skills but also appreciate its applications in everyday life. Whether you're a student or a professional, this knowledge is invaluable. (Mathematical Mastery, Everyday Applications)
What is the difference between inverse addition and subtraction?
+
Inverse addition specifically refers to finding the additive inverse of a number, while subtraction is the operation of finding the difference between two numbers. However, subtraction can be seen as the process of adding the inverse.
Can inverse addition be applied to negative numbers?
+
Yes, inverse addition applies to all real numbers, including negative numbers. For example, the additive inverse of (-4) is (4).
How is inverse addition used in real life?
+
Inverse addition is used in balancing budgets, solving equations in science, and simplifying mathematical problems in engineering and economics.



